Topics Covered
Introduction to Algebra Formula
Algebra is a branch of mathematics dealing with symbols and the rules for manipulating those symbols. It provides a way to represent and solve problems involving unknown values. Understanding algebraic formulas and their applications is essential for solving real-world problems across various fields, including science, engineering, and economics.

Key Algebra Formulas
Here are some fundamental algebra formulas that are widely used:
- Linear Equations:
- General form: ( ax + b = 0 )
- Slope-intercept form: ( y = mx + c )
- Where ( m ) is the slope and ( c ) is the y-intercept.
- Quadratic Equations:
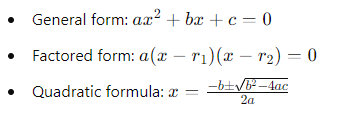
- Exponential Functions:
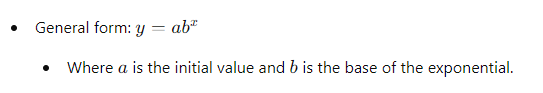
- Polynomials:
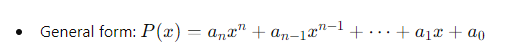
- Binomial Theorem:
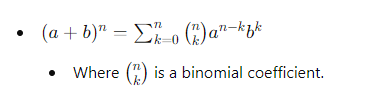
- Logarithmic Functions:

Real-World Examples of Algebra Formulas
1. Linear Equations in Economics:
- Problem: A company’s profit ( P ) depends on the number of units sold ( x ) according to the equation ( P = 50x – 2000 ).
- Solution: To find the break-even point (where profit is zero), set ( P = 0 ):

The company needs to sell 40 units to break even.
2. Quadratic Equations in Physics:
- Problem: The height ( h ) of a projectile at time ( t ) is given by ( h = -4.9t^2 + 20t + 5 ). When will the projectile hit the ground?
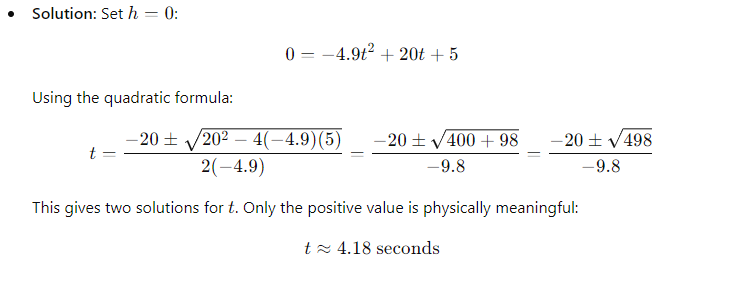
3. Exponential Functions in Biology:
- Problem: A bacteria population doubles every 3 hours. If the initial population is 100, find the population after 9 hours.
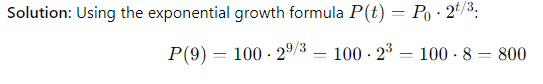
- The population after 9 hours is 800.
4. Polynomial Functions in Engineering:
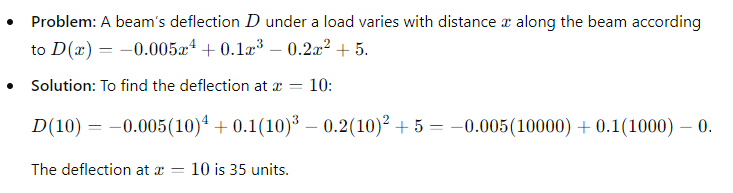
Frequently Asked Questions
Q1: What is the importance of algebra in everyday life?
A1: Algebra is important because it helps us solve problems involving unknown values, make predictions, and understand relationships between variables. It’s used in various fields like science, engineering, medicine, and economics.
Q2: How do linear equations apply in real life?
A2: Linear equations model relationships with a constant rate of change. They are used in budgeting, calculating distances, determining speeds, and predicting future events based on current trends.
Q3: What are some practical uses of quadratic equations?
A3: Quadratic equations are used to model projectile motion, optimize areas and volumes, determine profit maximization, and solve problems involving acceleration.
Q4: How are exponential functions used in the real world?
A4: Exponential functions model growth and decay processes such as population growth, radioactive decay, and compound interest calculations.
Q5: Why are polynomials important in engineering?
A5: Polynomials are used in engineering to model relationships and behaviors of systems, such as stress-strain curves, beam deflections, and electrical circuits.
Q6: How can I improve my understanding of algebra?
A6: Practice regularly, seek help from teachers or tutors, use online resources, work on real-life problems, and study algebraic concepts thoroughly to improve understanding.
By understanding these algebra formulas and their real-world applications, you can solve a wide range of problems more effectively and appreciate the relevance of algebra in everyday life.
